Aquasprite Theme Demo

follow and follow!!

Friday, 7 October 2011 , Posted by 12DEP11F1043 at 01:06



Equations for the transformation from Δ-load to Y-load 3-phase circuit

The general idea is to compute the impedance Ry at a terminal node of the Y circuit with impedances R', R'' to adjacent nodes in the Δ circuit by
R_y = \frac{R'R''}{\sum R_\Delta}
where RΔ are all impedances in the Δ circuit. This yields the specific formulae
R_1 = \frac{R_aR_b}{R_a + R_b + R_c},

R_2 = \frac{R_bR_c}{R_a + R_b + R_c},

R_3 = \frac{R_aR_c}{R_a + R_b + R_c}.

[edit]Equations for the transformation from Y-load to Δ-load 3-phase circuit

The general idea is to compute an impedance RΔ in the Δ circuit by
R_\Delta = \frac{R_P}{R_\mathrm{opposite}}
where RP = R1R2 + R2R3 + R3R1 is the sum of the products of all pairs of impedances in the Y circuit and Ropposite is the impedance of the node in the Y circuit which is opposite the edge with RΔ. The formula for the individual edges are thus
R_a = \frac{R_1R_2 + R_2R_3 + R_3R_1}{R_2},
R_b = \frac{R_1R_2 + R_2R_3 + R_3R_1}{R_3},
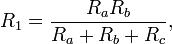
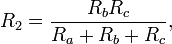
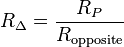
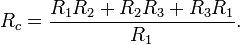
R_c = \frac{R_1R_2 + R_2R_3 + R_3R_1}{R_1}.

Currently have 0 comments:

Leave a Reply

Post a Comment